Read on Twitter
[THREAD: ZERO]
1/51
Once upon a time in the land of Mesopotamia, there lived a people who engaged in commerce, had a system of weights and measures, paid in currency, and most importantly, kept written records of all of this in clay tablets. These were the Sumerians.
1/51
Once upon a time in the land of Mesopotamia, there lived a people who engaged in commerce, had a system of weights and measures, paid in currency, and most importantly, kept written records of all of this in clay tablets. These were the Sumerians.
2/51
Writing was a real chore back then. The Sumerians would make tablets out of wet clay and use a hollow reed to etch circles and semi-circles into it while still wet and soft. Once done, the tablet would be baked in a kiln to make the etchings permanent. That's how they wrote.
Writing was a real chore back then. The Sumerians would make tablets out of wet clay and use a hollow reed to etch circles and semi-circles into it while still wet and soft. Once done, the tablet would be baked in a kiln to make the etchings permanent. That's how they wrote.
3/51
With time, the reed further evolved into a three-sided stylus. Instead of circles and semi-circles, the stylus did lines and wedges. We call those, cuneiform.
Unlike us, the Sumerians counted in 60s. We call this the sexagesimal system. Ours is in 10s, i.e. decimal.
With time, the reed further evolved into a three-sided stylus. Instead of circles and semi-circles, the stylus did lines and wedges. We call those, cuneiform.
Unlike us, the Sumerians counted in 60s. We call this the sexagesimal system. Ours is in 10s, i.e. decimal.
4/51
By around 2500 BC, the Akkadians entered the scene and drove the Sumerians out of Mesopotamia. What they didn't, however, is the cuneiform writing system. And the sexagesimal counting. By around 2000 BC, the system was mainstream all over Mesopotamia and Babylon.
By around 2500 BC, the Akkadians entered the scene and drove the Sumerians out of Mesopotamia. What they didn't, however, is the cuneiform writing system. And the sexagesimal counting. By around 2000 BC, the system was mainstream all over Mesopotamia and Babylon.
5/51
This is what cuneiform numbers looked like:
1 ๐น
2 ๐ซ
3 ๐
4 ๐
5 ๐
6 ๐
7 ๐
8 ๐
9 ๐
10 ๐
60 ๐น
If you notice, most of these symbols are made up of one or more wedges like this one: ๐น.
If you notice, the system isn't entirely in 60s but a mix of 10s and 60s.
This is what cuneiform numbers looked like:
1 ๐น
2 ๐ซ
3 ๐
4 ๐
5 ๐
6 ๐
7 ๐
8 ๐
9 ๐
10 ๐
60 ๐น
If you notice, most of these symbols are made up of one or more wedges like this one: ๐น.
If you notice, the system isn't entirely in 60s but a mix of 10s and 60s.
6/51
Also note that 60 looks identical to 1, both are represented by ๐น. We'll come to that a little later. First, let's write a few numbers in cuneiform to better understand the system. It's all a combination of wedges (๐น) and hooks (๐) in different permutations.
Also note that 60 looks identical to 1, both are represented by ๐น. We'll come to that a little later. First, let's write a few numbers in cuneiform to better understand the system. It's all a combination of wedges (๐น) and hooks (๐) in different permutations.
7/51
One last thing before we finally get down to writing numbers, don't let the symbols for 4-9 confuse you. They're just neatly arranged repetitions of ๐น. So, 4 is actually ๐น๐น๐น๐น rendered as ๐ to save space. Similarly, 9 is ๐น๐น๐น๐น๐น๐น๐น๐น๐น contracted to ๐.
One last thing before we finally get down to writing numbers, don't let the symbols for 4-9 confuse you. They're just neatly arranged repetitions of ๐น. So, 4 is actually ๐น๐น๐น๐น rendered as ๐ to save space. Similarly, 9 is ๐น๐น๐น๐น๐น๐น๐น๐น๐น contracted to ๐.
8/51
Let's start with the simplest number, 11. To the Sumerians, that'd be 10+1. 10 is ๐ and 1 is ๐น, so 11 becomes ๐๐น. Similarly, 15 would be 10+5, i.e. ๐๐. 20 becomes 10+10, i.e. ๐๐. 59 would be 10+10+10+10+10+9, or ๐๐๐๐๐๐.
Easy, no? But things change after 59.
Let's start with the simplest number, 11. To the Sumerians, that'd be 10+1. 10 is ๐ and 1 is ๐น, so 11 becomes ๐๐น. Similarly, 15 would be 10+5, i.e. ๐๐. 20 becomes 10+10, i.e. ๐๐. 59 would be 10+10+10+10+10+9, or ๐๐๐๐๐๐.
Easy, no? But things change after 59.
9/51
You see, from 1 thru 59, what we've done is count in 10s. Just like we do today. But the Sumerians, also counted in 60s. So they had a dedicated symbol for 60. It looked almost exactly like the symbol for 1, but significantly larger: ๐น. So, 60 wasn't ๐๐๐๐๐๐, but ๐น.
You see, from 1 thru 59, what we've done is count in 10s. Just like we do today. But the Sumerians, also counted in 60s. So they had a dedicated symbol for 60. It looked almost exactly like the symbol for 1, but significantly larger: ๐น. So, 60 wasn't ๐๐๐๐๐๐, but ๐น.
10/51
Again, the symbol for 60 looked similar to but was much larger than the symbol for 1. Since I cannot represent this size variation here, I will be representing 60 as ๐นs wherever the context isn't clear. Hope that helps.
Now let's try writing a few more numbers.
Again, the symbol for 60 looked similar to but was much larger than the symbol for 1. Since I cannot represent this size variation here, I will be representing 60 as ๐นs wherever the context isn't clear. Hope that helps.
Now let's try writing a few more numbers.
11/51
Take 63, for example. To the Sumerians, it'd be 60+3, i.e. ๐นs๐น๐น๐น. Just to reiterate, the first wedge here would be larger than the remaining three on an actual Mesopotamian tablet. 75, similarly, would be 60+10+5, i.e. ๐นs๐๐น๐น๐น๐น๐น.
Take 63, for example. To the Sumerians, it'd be 60+3, i.e. ๐นs๐น๐น๐น. Just to reiterate, the first wedge here would be larger than the remaining three on an actual Mesopotamian tablet. 75, similarly, would be 60+10+5, i.e. ๐นs๐๐น๐น๐น๐น๐น.
12/51
Finally, let's try a bigger number before moving on. How about 152? Let's see, we can break it down as 60+60+10+10+10+1+1 which gives us ๐นs๐นs๐๐๐๐น๐น. On a real tablet, of course, it'd appear as ๐น๐น๐๐๐๐น๐น with the first two wedges much bigger than the last two.
Finally, let's try a bigger number before moving on. How about 152? Let's see, we can break it down as 60+60+10+10+10+1+1 which gives us ๐นs๐นs๐๐๐๐น๐น. On a real tablet, of course, it'd appear as ๐น๐น๐๐๐๐น๐น with the first two wedges much bigger than the last two.
13/51
But this was problematic, the similarity of ๐นs and ๐น. Sizes could vary from hand to hand and a 62 (๐นs๐น๐น) could very easily be misread, or even mis-etched, as 3 (๐น๐น๐น)! This gave birth to the placeholder system. Someone decided to value a digit on where it sat.
But this was problematic, the similarity of ๐นs and ๐น. Sizes could vary from hand to hand and a 62 (๐นs๐น๐น) could very easily be misread, or even mis-etched, as 3 (๐น๐น๐น)! This gave birth to the placeholder system. Someone decided to value a digit on where it sat.
14/51
In our placeholder system, each positional shift to the left increases the value of the digit tenfold. We count as ones, tens, hundreds, and so on. The Sumerians counted as ones, 60s, 3,600s, and so on. Someone decided to make this a written standard.
In our placeholder system, each positional shift to the left increases the value of the digit tenfold. We count as ones, tens, hundreds, and so on. The Sumerians counted as ones, 60s, 3,600s, and so on. Someone decided to make this a written standard.
15/51
Now, the size of the wedge didn't matter. What mattered was its position. Thus, ๐น was 1 if in the ones (first) position, but 60 if in the sixties (second) position and 3,600 if in the third position. Just keep adding to the power of 60 as you move leftward.
Now, the size of the wedge didn't matter. What mattered was its position. Thus, ๐น was 1 if in the ones (first) position, but 60 if in the sixties (second) position and 3,600 if in the third position. Just keep adding to the power of 60 as you move leftward.
16/51
Thus, 152 could now be rendered as 60+60+10+10+10+1+1, i.e. ๐น๐น๐๐๐๐น๐น. But there's still one problem. In English, each digit has a single symbol so it's easy to tell its position in a number. Not so in cuneiform. There's no way to tell the positions apart.
Thus, 152 could now be rendered as 60+60+10+10+10+1+1, i.e. ๐น๐น๐๐๐๐น๐น. But there's still one problem. In English, each digit has a single symbol so it's easy to tell its position in a number. Not so in cuneiform. There's no way to tell the positions apart.
17/51
Take ๐น๐น๐๐๐๐น๐น, for instance. Is this 2 positions or 7? If it's 2, where do they break? The solution was to group symbols of each position together with spaces between adjacent groups. Thus, ๐น๐น๐๐๐๐น๐น became ๐น๐น ๐๐๐๐น๐น for better clarity.
Take ๐น๐น๐๐๐๐น๐น, for instance. Is this 2 positions or 7? If it's 2, where do they break? The solution was to group symbols of each position together with spaces between adjacent groups. Thus, ๐น๐น๐๐๐๐น๐น became ๐น๐น ๐๐๐๐น๐น for better clarity.
18/51
This tiny update revolutionized counting. Now, very large numbers could be rendered with both ease and clarity. Take 1,275, for instance:
21 sixties + (10+5), i.e. ๐๐๐น ๐๐.
This solved many problems, but not all. There are things positional counting didn't help with.
This tiny update revolutionized counting. Now, very large numbers could be rendered with both ease and clarity. Take 1,275, for instance:
21 sixties + (10+5), i.e. ๐๐๐น ๐๐.
This solved many problems, but not all. There are things positional counting didn't help with.
19/51
Take 120 and 2. 120 is ๐น๐น (2 sixties). 2 is also ๐น๐น (2 ones). How do you tell the difference? One solution is to draw from the context which is what the Babylonians did for centuries. ๐น๐น given as the population of a village is more likely to be 120 than 2.
Take 120 and 2. 120 is ๐น๐น (2 sixties). 2 is also ๐น๐น (2 ones). How do you tell the difference? One solution is to draw from the context which is what the Babylonians did for centuries. ๐น๐น given as the population of a village is more likely to be 120 than 2.
20/51
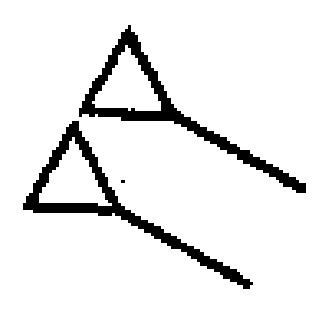
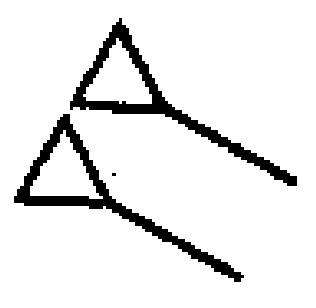
But there's got to be a way out of this ambiguity. Finally after centuries of research, some Babylonian genius came up with another groundbreaking idea: the slanting wedge. Two slanting wedges, to be precise. I'm gonna represent this with a ๐ but it looked more like this:
But there's got to be a way out of this ambiguity. Finally after centuries of research, some Babylonian genius came up with another groundbreaking idea: the slanting wedge. Two slanting wedges, to be precise. I'm gonna represent this with a ๐ but it looked more like this:
21/51
So, while the blanks stayed as placeholder separator, the new symbol ๐ came to mean "nothing in this position." Thus, ๐น ๐ was (1ร60)+(5ร1) = 65. But ๐น ๐ ๐ was (1ร3,600)+(0ร60)+(5ร1) = 3,605.
Today, we use a different symbol for the job, we call it zero.
So, while the blanks stayed as placeholder separator, the new symbol ๐ came to mean "nothing in this position." Thus, ๐น ๐ was (1ร60)+(5ร1) = 65. But ๐น ๐ ๐ was (1ร3,600)+(0ร60)+(5ร1) = 3,605.
Today, we use a different symbol for the job, we call it zero.
22/51
This should've also resolved the 120-2 dilemma. While both 120 and 2 were rendered as two wedges each (larger for 120 and smaller for 2) earlier, now 2 could be ๐น๐น (2 ones) and 120, ๐น๐น๐ (2 sixties plus zero ones).
But for some strange reason, this didn't happen.
This should've also resolved the 120-2 dilemma. While both 120 and 2 were rendered as two wedges each (larger for 120 and smaller for 2) earlier, now 2 could be ๐น๐น (2 ones) and 120, ๐น๐น๐ (2 sixties plus zero ones).
But for some strange reason, this didn't happen.
23/51
That's because the Babylonians, despite having invented the gamechanger of a symbol, never used it at the end of a number. Why? We don't know. So, 2 was still ๐น๐น, as was 120, and the Babylonians still had to tell the two apart from context and nothing else.
That's because the Babylonians, despite having invented the gamechanger of a symbol, never used it at the end of a number. Why? We don't know. So, 2 was still ๐น๐น, as was 120, and the Babylonians still had to tell the two apart from context and nothing else.
24/51
This leap to a terminal zero-marker usage would take centuries. That being said, it's impossible to overstate the impact the Babylonian zero-marker, even in its non-terminal usage, had on their commerce in particular and life in general.
This leap to a terminal zero-marker usage would take centuries. That being said, it's impossible to overstate the impact the Babylonian zero-marker, even in its non-terminal usage, had on their commerce in particular and life in general.
25/51
One last thing before we leave Babylon: the zero-marker wasn't always ๐. Different scribes used different "standards." Bรชl-bรขn-aplu, a 700 BC Babylonian, for instance, used 3 hooks (๐๐๐) to represent zero. I know, it looks like 30, but that's how he rolled.
One last thing before we leave Babylon: the zero-marker wasn't always ๐. Different scribes used different "standards." Bรชl-bรขn-aplu, a 700 BC Babylonian, for instance, used 3 hooks (๐๐๐) to represent zero. I know, it looks like 30, but that's how he rolled.
26/51
So it's clear, the Babylonians were using some kind of a proto-zero, if you will, at least 3,000 years ago if not earlier still. Sure, it needed tons of refinement, but a zero it was. But were they the first in the world to have done it? Maybe, maybe not.
So it's clear, the Babylonians were using some kind of a proto-zero, if you will, at least 3,000 years ago if not earlier still. Sure, it needed tons of refinement, but a zero it was. But were they the first in the world to have done it? Maybe, maybe not.
27/51
Turns out, the Egyptians were doing their own thing with numbers much before the Babylonians, possibly even the Sumerians. Sure, they used hieroglyphs much of which remains undeciphered to this day, but their number system was surprisingly closer to what we have today.
Turns out, the Egyptians were doing their own thing with numbers much before the Babylonians, possibly even the Sumerians. Sure, they used hieroglyphs much of which remains undeciphered to this day, but their number system was surprisingly closer to what we have today.
28/51
For one, theirs was a base 10 system, just like ours. In contrast, the Sumerians were base 60. We still use base 60, by the way, in certain niches which is why we have 60 seconds to a minute and 60 minutes to an hour. But base 10 is pretty much the mainstream.
For one, theirs was a base 10 system, just like ours. In contrast, the Sumerians were base 60. We still use base 60, by the way, in certain niches which is why we have 60 seconds to a minute and 60 minutes to an hour. But base 10 is pretty much the mainstream.
29/51
I could do another 30 tweets on the Egyptian counting system but won't. Instead, I'll spare you the details and come straight to the most startling of all things: The Egyptian zero.
It's called nfr.
That's Egyptian for good or beautiful and looks like this:
I could do another 30 tweets on the Egyptian counting system but won't. Instead, I'll spare you the details and come straight to the most startling of all things: The Egyptian zero.
It's called nfr.
That's Egyptian for good or beautiful and looks like this:
30/51
If this letter sounds like Nefertiti, that's because the name's derived off it. She was famous as one of the most beautiful queens Ancient Egypt ever had. Anyway, the point of import here is that the Egyptians had a zero at least as early as 1770 BC.
If this letter sounds like Nefertiti, that's because the name's derived off it. She was famous as one of the most beautiful queens Ancient Egypt ever had. Anyway, the point of import here is that the Egyptians had a zero at least as early as 1770 BC.
31/51
Between the Egyptians and the Babylonians, it's hard to tell who came up with the zero-marker, however rudimentary, first. That said, most evidences available point to the Egyptians. Nfr can be seen as human civilization's first attempt at denoting "nothing."
Between the Egyptians and the Babylonians, it's hard to tell who came up with the zero-marker, however rudimentary, first. That said, most evidences available point to the Egyptians. Nfr can be seen as human civilization's first attempt at denoting "nothing."
32/51
But the idea of expressing nothingness didn't remain alien to other parts of the world for long. By 400 BC, the Mayans were counting using pebbles and stick in what's considered the most economical number system ever invented โ base 20. Guess what, they had a zero too!
But the idea of expressing nothingness didn't remain alien to other parts of the world for long. By 400 BC, the Mayans were counting using pebbles and stick in what's considered the most economical number system ever invented โ base 20. Guess what, they had a zero too!
33/51
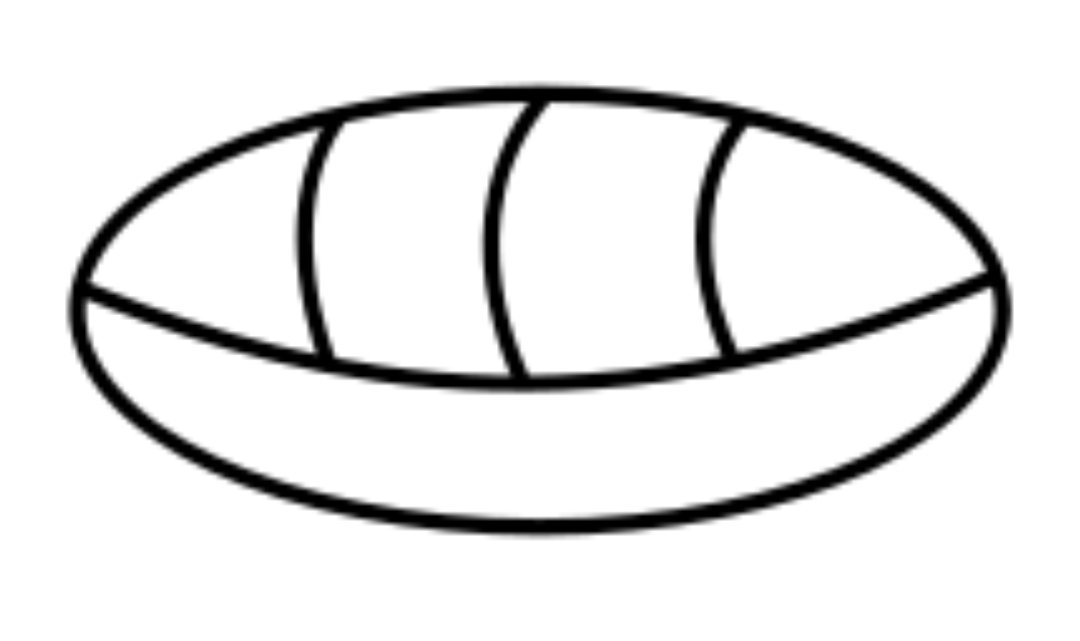
The Mayans used a system of pebbles and sticks to write their numbers. Pebbles were later written as dots, sticks as bars. Dots represented 1 and bars represented 5. After 19, they needed a zero to write 20. So they brought in the shell. This is how it looked:
The Mayans used a system of pebbles and sticks to write their numbers. Pebbles were later written as dots, sticks as bars. Dots represented 1 and bars represented 5. After 19, they needed a zero to write 20. So they brought in the shell. This is how it looked:
34/51
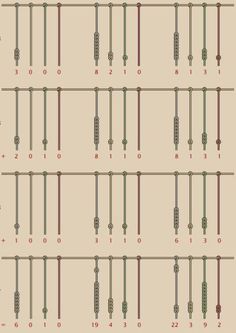
The Incas of South America, too, had a base 10 system as early as 2000 BC. But they weren't into writing. Instead, they had a system of strings with knots called quipu to represent different numerals. A knotless string represented nothing, i.e. zero.
The Incas of South America, too, had a base 10 system as early as 2000 BC. But they weren't into writing. Instead, they had a system of strings with knots called quipu to represent different numerals. A knotless string represented nothing, i.e. zero.
35/51
Coming back to the Old World, the first Europeans with some kind of number system were the Greeks. They had a positional lettering system in base 10, sometimes also 5, which is what the Romans would borrow later. But the Greeks has no zero, at least not until Alexander.
Coming back to the Old World, the first Europeans with some kind of number system were the Greeks. They had a positional lettering system in base 10, sometimes also 5, which is what the Romans would borrow later. But the Greeks has no zero, at least not until Alexander.
36/51
The Greeks did have trouble counting and there was too much dependency on context, but they had no idea zero could solve this problem. Not until 331 BC. That's when Alexander invaded Babylon and learned of this wonderful new notation that represented nothing.
The Greeks did have trouble counting and there was too much dependency on context, but they had no idea zero could solve this problem. Not until 331 BC. That's when Alexander invaded Babylon and learned of this wonderful new notation that represented nothing.
37/51
But the Greeks wrote zero the way we do today: as a circle. There's several theories around how two tiled wedges of Babylon turned into the Greek circle. The most widely accepted is that the circle is omicron. It's the first letter of ฮฟแฝฮดฮญฮฝ, Greek for nothing.
But the Greeks wrote zero the way we do today: as a circle. There's several theories around how two tiled wedges of Babylon turned into the Greek circle. The most widely accepted is that the circle is omicron. It's the first letter of ฮฟแฝฮดฮญฮฝ, Greek for nothing.
38/51
The first time zero makes an appearance in Asia is at the most generous least 100 years after the Greeks started with the circle notation. In fact, it can all be narrowed down to one individual.
Pingala.
You probably expected the name ฤryabhatta, but that's much later.
The first time zero makes an appearance in Asia is at the most generous least 100 years after the Greeks started with the circle notation. In fact, it can all be narrowed down to one individual.
Pingala.
You probably expected the name ฤryabhatta, but that's much later.
39/51
Pingala is said to have come up with a primitive form of the binary system in his 200 BC work Chandaแธฅลฤstra. This is the text that introduces us to ลลซnya, a dot notation that's understood to represent nothingness. As a notation, this is the first Asian zero.
Pingala is said to have come up with a primitive form of the binary system in his 200 BC work Chandaแธฅลฤstra. This is the text that introduces us to ลลซnya, a dot notation that's understood to represent nothingness. As a notation, this is the first Asian zero.
40/51
But Pingala's zero isn't really the zero we know today. It didn't serve any mathematical function and was just a symbolic representation for nothingness. Even his binary system was not ลลซnya (0) and one, but laghu and guru.
But Pingala's zero isn't really the zero we know today. It didn't serve any mathematical function and was just a symbolic representation for nothingness. Even his binary system was not ลลซnya (0) and one, but laghu and guru.
41/51
The next big exhibit comes from, by the most liberal of estimates, around 224 AD. It's a Sanskrit text written in the ลฤradฤ script (a 3rd generation descendant of Brahmi) on a piece of birch bark that was discovered in Bakhshali near Peshawar in 1881.
The next big exhibit comes from, by the most liberal of estimates, around 224 AD. It's a Sanskrit text written in the ลฤradฤ script (a 3rd generation descendant of Brahmi) on a piece of birch bark that was discovered in Bakhshali near Peshawar in 1881.
42/51
ฤryabhatta is erroneously named by most Indian historians as the "inventor" of zero but truth is, he doesn't show up at least until the 500 AD, a good 250 years after the Bakhshali manuscript.
ฤryabhatta's system, just like Pingala's, doesn't have a numerical zero.
ฤryabhatta is erroneously named by most Indian historians as the "inventor" of zero but truth is, he doesn't show up at least until the 500 AD, a good 250 years after the Bakhshali manuscript.
ฤryabhatta's system, just like Pingala's, doesn't have a numerical zero.
43/51
ฤryabhatta's kha (his name for zero) and Pingala's ลลซnya were both mere symbolic. They served no numerical functions although they did aid calculations, just as placeholder variables aid calculations today.
ฤryabhatta's kha (his name for zero) and Pingala's ลลซnya were both mere symbolic. They served no numerical functions although they did aid calculations, just as placeholder variables aid calculations today.
44/51
Of course there's also debate on whether ฤryabhatta even existed and if he did, whether as one individual or many. But that's a debate we won't get into here and just work with the assumption that he existed and as a single individual.
Of course there's also debate on whether ฤryabhatta even existed and if he did, whether as one individual or many. But that's a debate we won't get into here and just work with the assumption that he existed and as a single individual.
45/51
About half a century after ฤryabhatta, comes Varฤhamihira of Ujjain. He, like his predecessors, didn't have a zero in any numerical sense but he gave it a new name, ฤkฤลa. The word is also Sanskrit for sky. Do now we have ลลซnya, kha, and ฤkฤลa โ all mere notational.
About half a century after ฤryabhatta, comes Varฤhamihira of Ujjain. He, like his predecessors, didn't have a zero in any numerical sense but he gave it a new name, ฤkฤลa. The word is also Sanskrit for sky. Do now we have ลลซnya, kha, and ฤkฤลa โ all mere notational.
46/51
One of the biggest medieval evidence against India's authority over zero comes from Severus Sebokht, a Syrian scholar and bishop who taught at the Theological School of Nisibis. He was also the first Syrian to mention the Indian number system; as an admirer, no less.
One of the biggest medieval evidence against India's authority over zero comes from Severus Sebokht, a Syrian scholar and bishop who taught at the Theological School of Nisibis. He was also the first Syrian to mention the Indian number system; as an admirer, no less.
47/51
In 662 AD, this Syrian fan of Indian arithmetic wrote about how the Indians expressed numbers using nine symbols.
Nine, not ten.
That's one of the most compelling arguments against the Indian zero as a marker of numerical significance.
http://www.tertullian.org/fathers/severus_sebokht_constellations_01_intro.htm
In 662 AD, this Syrian fan of Indian arithmetic wrote about how the Indians expressed numbers using nine symbols.
Nine, not ten.
That's one of the most compelling arguments against the Indian zero as a marker of numerical significance.
http://www.tertullian.org/fathers/severus_sebokht_constellations_01_intro.htm
48/51
So who gets to own zero exactly?
The Babylonians who invented the idea of expressing numerically significant nothingness with two slanting wedges around 3,000 years ago?
The Egyptians who repurposed nfr, the hieroglyph for beautiful, to denote nothingness?
So who gets to own zero exactly?
The Babylonians who invented the idea of expressing numerically significant nothingness with two slanting wedges around 3,000 years ago?
The Egyptians who repurposed nfr, the hieroglyph for beautiful, to denote nothingness?
49/51
The Mayans who first wrote it as an oval?
The Greeks who first wrote it as a circle?
The Indians who first wrote it as a dot but with no numerical significance?
Truth is, it belongs to all. Mathematics, like sciences, is a trans-civilizational endeavor.
The Mayans who first wrote it as an oval?
The Greeks who first wrote it as a circle?
The Indians who first wrote it as a dot but with no numerical significance?
Truth is, it belongs to all. Mathematics, like sciences, is a trans-civilizational endeavor.
50/51
No one civilization can claim monopoly over something as broad as zero. Different improvisations emerged in different parts of the world as and when the needs were felt. There's little value, then, in romanticing a fictitious antiquity where one culture owns it all.
No one civilization can claim monopoly over something as broad as zero. Different improvisations emerged in different parts of the world as and when the needs were felt. There's little value, then, in romanticing a fictitious antiquity where one culture owns it all.
51/51
Funnily enough, as if to close the loop, this zero that India inherited from Pingala, made its way back into Europe as part of the Hindu-Arabic system in the 11th century thanks to the Moors of Andalusia who were voracious translators.
That's how zero came to be.
Funnily enough, as if to close the loop, this zero that India inherited from Pingala, made its way back into Europe as part of the Hindu-Arabic system in the 11th century thanks to the Moors of Andalusia who were voracious translators.
That's how zero came to be.
