With friends at LSE, I recently had a reading group session in memory of Emmanuel Farhi. I discussed some of his influential papers on production networks with David Baqaee.
I thought I'd summarise this discussion here for people interested in this beautiful line of work
I thought I'd summarise this discussion here for people interested in this beautiful line of work

Out of the many papers Baqaee & Farhi wrote, 3 are especially inspirational. They are in yellow below:
Paper 1. lays the foundation of their theory of input-output networks
Paper 2. applies it to inefficient economies (=with markups)
Paper 1. lays the foundation of their theory of input-output networks
Paper 2. applies it to inefficient economies (=with markups)
Paper 3. shows that the structure of an economy's production network matters a great deal for the amplification of shocks.
Even shocks to only one firm or sector.
And even in a perfectly competitive economy.
Even shocks to only one firm or sector.
And even in a perfectly competitive economy.
But why are production networks so important?
They are super useful to link micro and macro. We can only hope to build and aggregate pro function from firms' individual optimisation problems if we know something about the complex web of trade relationships that unites them.
They are super useful to link micro and macro. We can only hope to build and aggregate pro function from firms' individual optimisation problems if we know something about the complex web of trade relationships that unites them.
Also, the deluge of good-quality firm-level microdata in recent years presses us to use a reliable theory of aggregation.
See below for a short summary of the motivation of this research agenda.
See below for a short summary of the motivation of this research agenda.
Aggregation is actually a very old problem in Economics, and an interesting chapter of the history of economic thought.
It dates back to the 60s, with the "Cambridge-Cambridge controversy" about whether it even makes sense talk about aggregate capital and aggregate prod function
It dates back to the 60s, with the "Cambridge-Cambridge controversy" about whether it even makes sense talk about aggregate capital and aggregate prod function
B&F revived this debate in their paper 1.
If interested, this beautiful presentation of the paper by Emmanuel Farhi is really worth watching.
If interested, this beautiful presentation of the paper by Emmanuel Farhi is really worth watching.
In this paper, B&F build an aggregate production function from the ground up i.e. completely microfounding it as a result of endogenous decisions.
Their framework is very general:
- arbitrary network linkages
- arbitrary elasticities of subs btw factors
- arbitrary factors
Their framework is very general:
- arbitrary network linkages
- arbitrary elasticities of subs btw factors
- arbitrary factors
This disaggregated framework can then be used to analyse the spread of productivity shocks to any firm, or the spread of demand shocks for any good.
It allows for complex substitution and reallocation patterns.
It allows for complex substitution and reallocation patterns.
Before B&F, much of the production network literature used a multi-input Cobb-Douglas production function to describe how a firm's output (y_i) depends on inputs produced by other firms (x_ij).
Pros: neat and tractable
Cons: strong constraints on propagation of shocks
Pros: neat and tractable
Cons: strong constraints on propagation of shocks
In particular, Cobb-Douglas imposes constant factor shares. This is woefully inadequate to study reallocation in an input-output economy.
This is deeply problematic for macroeconomists interested in relating in markups to
in markups to  in the labour share for instance.
in the labour share for instance.
This is deeply problematic for macroeconomists interested in relating
 in markups to
in markups to  in the labour share for instance.
in the labour share for instance.
To see how important it is to depart from CB, consider the following example (in Carvalho & Tahbaz-Salehi, 2019).
Firm k indirectly buys from i and j. The consequences of a decrease in productivity of j on i are very different in the two networks below.
Firm k indirectly buys from i and j. The consequences of a decrease in productivity of j on i are very different in the two networks below.
In (a), i's output decreases. In (b), i's output increases.
In both cases, k substitutes away from the branch using j's output.
In both cases, k substitutes away from the branch using j's output.
So two things interact in complex ways when shocks propagate on production networks:
- Elasticities of substitution at each node (=firm)
- The structure of the production network
Developing a general theory of shocks on networks is one of B&F's most impressive achievements.
- Elasticities of substitution at each node (=firm)
- The structure of the production network
Developing a general theory of shocks on networks is one of B&F's most impressive achievements.
In "Productivity and Misallocation", B&F extend their model to inefficient economies.
They use it to decompose TFP growth into pure technology improvements and reallocation of factors toward more productive firms. This paper is one of the most interesting I have ever read!
They use it to decompose TFP growth into pure technology improvements and reallocation of factors toward more productive firms. This paper is one of the most interesting I have ever read!
Importantly, the second term in the equation above is 0 in efficient economies.
This is a consequence of the envelope theorem: if the allocation of inputs was optimally determined to start with, small changes in prod would not affect the allocation (dX=0)
This is a consequence of the envelope theorem: if the allocation of inputs was optimally determined to start with, small changes in prod would not affect the allocation (dX=0)
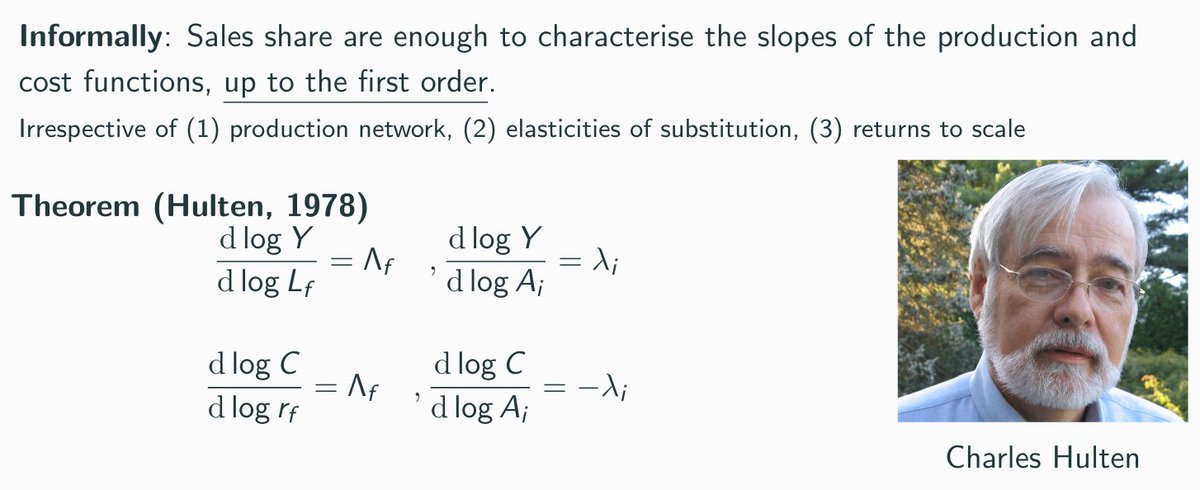
This persistence in allocation in efficient economies is what underpins a remarkable aggregation result: Hulten's theorem.
It says that sales or factor shares (small and big lambda resp.) are enough to describe the slope of any aggregate prod function, up to the first order
It says that sales or factor shares (small and big lambda resp.) are enough to describe the slope of any aggregate prod function, up to the first order
However, Hulten's theorem breaks in two important cases:
- Inefficient economies (paper 2)
- Second order, that is nonlinearities in the production function (paper 3.)
B&F provide formulas describing aggregate production functions in these two cases. Another massive contribution
- Inefficient economies (paper 2)
- Second order, that is nonlinearities in the production function (paper 3.)
B&F provide formulas describing aggregate production functions in these two cases. Another massive contribution
Taking their formulas to the data, they track the sources of growth in the US over the last 20 years, paying particular attention to the role of misallocation due to firms' market power.
The result? Pure technology growth accounts for only half of the aggregate Solow residual.
The rest is due to reshuffling resources around and distributing them to the most efficient parts of the economy.
The rest is due to reshuffling resources around and distributing them to the most efficient parts of the economy.
This echoes a result from recent papers on US markups: market power is not increasing because markups are increasing for all firms, but because firms with higher markups have grown bigger.
In their case, big firms with potentially high markups also concentrate more resources.
In their case, big firms with potentially high markups also concentrate more resources.
The last paper, "the Micro origins of Macro shocks" looks at something fundamental to understand the economic consequences of Covid-19 (among other crises): how shocks to parts of the economy interact with other shocks.
Do they amplify, or mitigate each other?
Do they amplify, or mitigate each other?
Going beyond the first-order result of Hulten matters massively here. And both the elasticities of substitution and the network topology will affect the second derivative of the aggregate production function.
See the simplified example below (from one of B&F's Covid papers)
See the simplified example below (from one of B&F's Covid papers)
Their results show us why the intuition of Hulten's theorem is fragile. Shocks to essential (=low elasticity) and pervasive (=connected) parts of the economy like energy have consequences beyond what the sales shares of these sectors could lead us to believe.
This is only a partial and subjective overview of Baqaee & Farhi's contributions. But it hopefully gives an idea of why their ideas matter so much. And why we have such a big intellectual debt toward Emmanuel Farhi.
These slides have more details: https://www.dropbox.com/s/mxavd61fyfjvt9c/Reading_group_Emmanuel_Farhi_s_late_work.pdf?dl=0
These slides have more details: https://www.dropbox.com/s/mxavd61fyfjvt9c/Reading_group_Emmanuel_Farhi_s_late_work.pdf?dl=0

 Read on Twitter
Read on Twitter